You may often encounter questions involving motion in a lift. Generally such questions are simple, but you should have no confusion in this context. So keep the following points in mind:
The weight of a body of mass ‘m’ in a lift can be remembered as m(g-a) in all situations if you apply the proper sign to the acceleration ‘a’ of the lift. The acceleration due to gravity ‘g’ always acts vertically downwards and its sign is taken as positive. The following cases can arise in this connection:
(1) Lift moving down with acceleration of magnitude ‘a’:
In this case ‘a’ also is positive and the weight is m(g-a) which is less than the real weight of the body (when it is at rest).
(2) Lift moving up with acceleration:
In this case ‘a’ is negative and the weight is m[g-(-a)] = m(g+a).
(3) Lift moving down with retardation (going to stop while moving down):
In this case also ‘a’ is negative and the weight is m[g-(-a)] = m(g+a) which is greater than the actual weight.
(4) Lift moving up with retardation (going to stop while moving up):
In this case ‘a’ is positive and the weight is m(g-a)
(5) Lift moving up or down with uniform velocity:
In this case ‘a’ is zero and the weight is mg.
(6) Lift moving down with acceleration of magnitude ‘g’ (falling freely under gravity as is the case when the rope carrying the lift breaks):
In this case ‘a’ is positive and the weight is m(g-g) which is zero.
Let us now consider the following question: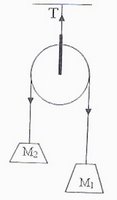
The weight of a body of mass ‘m’ in a lift can be remembered as m(g-a) in all situations if you apply the proper sign to the acceleration ‘a’ of the lift. The acceleration due to gravity ‘g’ always acts vertically downwards and its sign is taken as positive. The following cases can arise in this connection:
(1) Lift moving down with acceleration of magnitude ‘a’:
In this case ‘a’ also is positive and the weight is m(g-a) which is less than the real weight of the body (when it is at rest).
(2) Lift moving up with acceleration:
In this case ‘a’ is negative and the weight is m[g-(-a)] = m(g+a).
(3) Lift moving down with retardation (going to stop while moving down):
In this case also ‘a’ is negative and the weight is m[g-(-a)] = m(g+a) which is greater than the actual weight.
(4) Lift moving up with retardation (going to stop while moving up):
In this case ‘a’ is positive and the weight is m(g-a)
(5) Lift moving up or down with uniform velocity:
In this case ‘a’ is zero and the weight is mg.
(6) Lift moving down with acceleration of magnitude ‘g’ (falling freely under gravity as is the case when the rope carrying the lift breaks):
In this case ‘a’ is positive and the weight is m(g-g) which is zero.
Let us now consider the following question:

A light, frictionless pulley is suspended from the ceiling using a light string. Another light string is passed over the pulley. If unequal masses M1 and M2 (M1 >M2) are attached to the ends of this string and the system is released, what will be tension ‘T’ in the string that carries the pulley?
(a) (M1+M2)g (b) (M1- M2)g (c) M1M2g/(M1+M2) (d) 2M1M2g/(M1+M2) (e) 4M1M2g/(M1+M2)
(a) (M1+M2)g (b) (M1- M2)g (c) M1M2g/(M1+M2) (d) 2M1M2g/(M1+M2) (e) 4M1M2g/(M1+M2)
The tension in the string that caries the pulley is due to the weights of the masses m1 and m2. But these weights are not M1g and M2g but M1(g-a) and M2(g+a) because M1 is moving down with acceleration ‘a’ and M2 is moving up with acceleration ‘a’. The magnitude of ‘a’ is given by
a = Net driving force/ Total mass moved = (M1- M2)g/(M1+M2)
Therefore, tension, T = M1(g-a) + M2(g+a) = 4M1M2g/(M1+M2) on substituting for ‘a’. The correct option is (e)
Now consider the following simple question:
A man of mass 70 kg records his weight on a weighing scale placed inside a lift. The ratio of the weight of the man recorded when the lift is ascending with a uniform speed of 1m/s to the weight recorded when the lift is descending with a uniform speed of 2m/s will be
(a) 2 (b) 0.5 (c) 3 (d) 1.5 (e) 1
You should note that his weight is the same as his real weight (when he is at rest). There is no distinction between state of rest and uniform motion. To put this in a different manner, the acceleration of the lift is zero and hence his weight is unchanged. The ratio of the weights is therefore equal to unity [Option (e)].
Now consider the following MCQ:
What is the minimum acceleration with which a fire man can slide down a rope whose breaking strength is 80% of his weight?
(a) 0.2g (b) 0.1g (c) ) 0.9g (d) g (e) 1.1g
This is a situation where the fire man has a rope of insufficient strength. He wants to come down from an unsafe height. He can use the rope to slide down with acceleration so that his weight is reduced (as in a lift moving down with acceleration). His minimum acceleration ‘a’ is given by
m(g-a) = 0.8mg
We have equated his reduced weight to the breaking strength of the rope. The value of ‘a’ is 0.2g [Option (a)]. Note that the rope will break if the acceleration of the fire man is less than this and also that he can use a weaker rope if his acceleration is greater than this.
a = Net driving force/ Total mass moved = (M1- M2)g/(M1+M2)
Therefore, tension, T = M1(g-a) + M2(g+a) = 4M1M2g/(M1+M2) on substituting for ‘a’. The correct option is (e)
Now consider the following simple question:
A man of mass 70 kg records his weight on a weighing scale placed inside a lift. The ratio of the weight of the man recorded when the lift is ascending with a uniform speed of 1m/s to the weight recorded when the lift is descending with a uniform speed of 2m/s will be
(a) 2 (b) 0.5 (c) 3 (d) 1.5 (e) 1
You should note that his weight is the same as his real weight (when he is at rest). There is no distinction between state of rest and uniform motion. To put this in a different manner, the acceleration of the lift is zero and hence his weight is unchanged. The ratio of the weights is therefore equal to unity [Option (e)].
Now consider the following MCQ:
What is the minimum acceleration with which a fire man can slide down a rope whose breaking strength is 80% of his weight?
(a) 0.2g (b) 0.1g (c) ) 0.9g (d) g (e) 1.1g
This is a situation where the fire man has a rope of insufficient strength. He wants to come down from an unsafe height. He can use the rope to slide down with acceleration so that his weight is reduced (as in a lift moving down with acceleration). His minimum acceleration ‘a’ is given by
m(g-a) = 0.8mg
We have equated his reduced weight to the breaking strength of the rope. The value of ‘a’ is 0.2g [Option (a)]. Note that the rope will break if the acceleration of the fire man is less than this and also that he can use a weaker rope if his acceleration is greater than this.
No comments:
Post a Comment